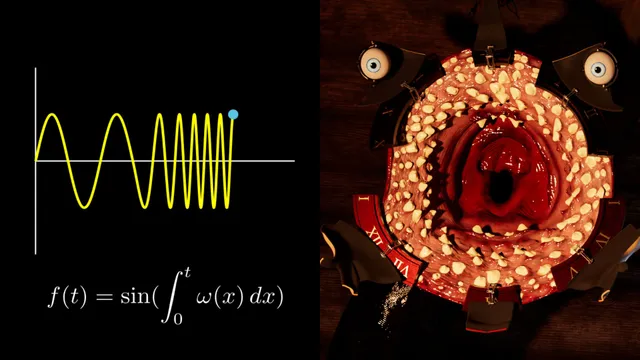
Variable Rate Function Traversal (Or: How to Make Cartoons Go Faster)
A method for changing the rate at which a function is traversed over time and how it's useful for 3D animation.
Analytics
Comments
8.5
I may still be calibrating my grading, but this one stood out to me. Very good use of manim, BTW :-)
7.3
An idea of making mathematical content on github was interesting, clear explanation and easy to understand.
6.5
Animation techniques are definitely my "cup of tea", so I enjoyed this very much.
I found the following difficult to believe:
"The function f(t) = sin(ωt) is only able to model oscillating motion when ω is constant.."
but I am minded to entertain its truth, given how impressed I was by the author's entry.
Great work & thank you for sharing your expertise!
6.7
Good use of animations, concise writing. Thanks for sharing this with the world!
7.6
Great explanation and source code provided
5.1
I liked how the problem was introduced, and the solution felt very intuitive and natural to me. The visualizations are great.
4.7
Article has nice images and animations. The content is not that strong. IMO, integration is useless. The problem with the non integrated version of w(t) was its a discontinious function. That was the reason why the animation has speed jump. Integration made that function continious so the jump become smooth. If you started with a continious function such as a polynomial, you would have almost the same affect without integration. That may solve your performance problems, but since I have no experience on maya, I'm not sure about this.
8.7
Amazing, well explained! I really enjoyed it!